Merhaba Arkadaşlar ,
İzmir'de Bilgisayar Mühendisliğinde okuyorum.Lineer Cebir Ödevi verildi hocamız tarafından , ilk defa zorlandım 1.soruyu kolaylıkla yaptım ama 2,3,4,5.sorularda takıldım.Yardımlarınızı bekliyorum.Şimdiden Teşekkür ederim,saygılarımla..
----------------------------------------------------------------------------------
Q1. Determine whether the below given set V is closed under the usual matrix addition and usual scalar multiplication. V is the set of all 2x2 real matrices where a=d.
The (usual) matrix addition: .
The (usual) scalar multiplication: .
(The Problem 4 in Exercises 6.1, Page 278 of the text book by Kolman-Hill.)
A.1
For Matrix Addition ; Where a=d = a and d are always equal , so all 2x2 real matrices must be closed for matrix addition.Therefore , according to matrix addition it is closed under the usual matrix addition.
For Matrix Multiplication ; where a=d = , A and D are equal ( , so any k constant multiply with the matrices , its result will be same for a and d.Therefore it is always closed for scalar multiplication.
Q2. Determine whether or not the set of 3-dimensional real vectors together with the following vector addition and scalar multiplication operation constitutes a linear (vector) space over the real number set as the scalar field.
Vector addition: =. Scalar multiplication: :
A.2
For Vector Addition ; = X1,X2 and X3 are element of the vector (V).Y1 , Y2 and Y3 are scalar (F).Their result are gathering in R3 . R3 (3-dimensional real vector space )
if X1 and Y1 can be added and can be seen in the vector space.This matrix can be defined in R3 (R3 which means that it has there rows and it is 3-dimensional real vector space). As a result , The result of X and Y set arent satisfied Closureness specify.This means that , The result isnt in a vector space according to closureness.It is not closed.
For Scalar Multiplication , : , This means that
Q3. Determine whether or not the set of all positive real numbers u with the vector addition defined as u+v= uv and scalar multiplication defined as c·u= constitutes a linear (vector) space over the real number set as the scalar field. If it is not a vector space, list the properties that fail to hold.
(The Problem 17 in Exercises 6.1, Page 278 of the text book by Kolman-Hill.)
Where, uv denotes the real multiplication and is the cth power of u. Note that u+v is not the usual real addition: Use any other symbol instead of + if you feel uncomfortable with +.
Q4. Consider the unit square shown in the accompanying figure. Let W be the set of all vectors of the form , where 0≤x≤1, 0≤y≤1. That is the set of all vectors whose tail is at the origin and whose head is a point inside or on the square. Is W a subspace of R2? Explain.
(The Problem 4 in Exercises 6.2, Page 287 of the text book by Kolman-Hill.)
Q5. Which of the following subset of P2 (i.e. the set of second order real polynomials) are subspaces? The set of all polynomials of the form
a) a2t2+a1t+a0 where a1= a0=0. b) a2t2+a1t+a0 where a1= 2 a0. c) a2t2+a1t+a0 where a2+a1+a0=0
İzmir'de Bilgisayar Mühendisliğinde okuyorum.Lineer Cebir Ödevi verildi hocamız tarafından , ilk defa zorlandım 1.soruyu kolaylıkla yaptım ama 2,3,4,5.sorularda takıldım.Yardımlarınızı bekliyorum.Şimdiden Teşekkür ederim,saygılarımla..
----------------------------------------------------------------------------------
Q1. Determine whether the below given set V is closed under the usual matrix addition and usual scalar multiplication. V is the set of all 2x2 real matrices where a=d.
The (usual) matrix addition: .
The (usual) scalar multiplication: .
(The Problem 4 in Exercises 6.1, Page 278 of the text book by Kolman-Hill.)
A.1
For Matrix Addition ; Where a=d = a and d are always equal , so all 2x2 real matrices must be closed for matrix addition.Therefore , according to matrix addition it is closed under the usual matrix addition.
For Matrix Multiplication ; where a=d = , A and D are equal ( , so any k constant multiply with the matrices , its result will be same for a and d.Therefore it is always closed for scalar multiplication.
Q2. Determine whether or not the set of 3-dimensional real vectors together with the following vector addition and scalar multiplication operation constitutes a linear (vector) space over the real number set as the scalar field.
Vector addition: =. Scalar multiplication: :
A.2
For Vector Addition ; = X1,X2 and X3 are element of the vector (V).Y1 , Y2 and Y3 are scalar (F).Their result are gathering in R3 . R3 (3-dimensional real vector space )
if X1 and Y1 can be added and can be seen in the vector space.This matrix can be defined in R3 (R3 which means that it has there rows and it is 3-dimensional real vector space). As a result , The result of X and Y set arent satisfied Closureness specify.This means that , The result isnt in a vector space according to closureness.It is not closed.
For Scalar Multiplication , : , This means that
Q3. Determine whether or not the set of all positive real numbers u with the vector addition defined as u+v= uv and scalar multiplication defined as c·u= constitutes a linear (vector) space over the real number set as the scalar field. If it is not a vector space, list the properties that fail to hold.
(The Problem 17 in Exercises 6.1, Page 278 of the text book by Kolman-Hill.)
Where, uv denotes the real multiplication and is the cth power of u. Note that u+v is not the usual real addition: Use any other symbol instead of + if you feel uncomfortable with +.
Q4. Consider the unit square shown in the accompanying figure. Let W be the set of all vectors of the form , where 0≤x≤1, 0≤y≤1. That is the set of all vectors whose tail is at the origin and whose head is a point inside or on the square. Is W a subspace of R2? Explain.
(The Problem 4 in Exercises 6.2, Page 287 of the text book by Kolman-Hill.)
Q5. Which of the following subset of P2 (i.e. the set of second order real polynomials) are subspaces? The set of all polynomials of the form
a) a2t2+a1t+a0 where a1= a0=0. b) a2t2+a1t+a0 where a1= 2 a0. c) a2t2+a1t+a0 where a2+a1+a0=0
İlk önce türkçeye çevirmek lazım tabi.
İlk önce türkçeye çevirmek lazım tabi.
Efendim Çok sıkışık durumdayım , bütün notlarım tam , Lineer Cebir dersinde Determinantlara kadar hep iyi notlar aldım , Nezaman Vektörlere geldik batırmaya başladım , sizin verceğiniz cevaplar benimkonuyu anlamamada yardımcı olucak.Size çok dua edicem , Anlmadığınız yerleri söyleyin ben türkçeye çeviririm efendim size.
1.Soruyu yapmanıza gerek yok ben hallettim efendim
SORULARIN TÜRKÇELERİ BURDA MEVCUTTUR TEKRAR TEŞEKKÜR EDERİM , ACİL OLARAK CUMA GÜNÜ VERMEM GEREK LÜTFEN YARDIM EDİN.
2.Soru
Burada bizden vector adition ozelliklerini tektek kontrol etmemızı ıstıyor
Ilk sekılde yanı 5 tane ozellıgı tek tek kontrol edeceksın ,scalar multıplıcatıon
da ıse 5 olan sm ozellıklerını tektek kullanarak bunun lınear space olup olmadıgını
kontrol edeceksın
3.soru
Burada bızden normal olan toplama ıslemını u+v yerıne uv seklınde tanımlayarak
Toplama ıslemının 5 ozellıgını saglayıp saglamadıgını kontrol etmemızı sonra 5 tane ca
Maya gore kontrol etmemızı ıstıyo sonra uc yide scalar multıplıcatıona gore kontrol
Etmemız ıstenıyor
4.soru
Bizim rkare deki bu sekılın ıcındekı 0 1 arasındakı x ve 0 bır arasındakı y nın altuzay olup olmadıgını
Kontrol etmemıızı ıstıyor
5.soru
Verılen polınomların subspace ( alt uzay ) olup olmadıgını tek tek gostermemizi istiyor bizden.
SORULARIN TÜRKÇELERİ BURDA MEVCUTTUR TEKRAR TEŞEKKÜR EDERİM , ACİL OLARAK CUMA GÜNÜ VERMEM GEREK LÜTFEN YARDIM EDİN.
2.Soru
Burada bizden vector adition ozelliklerini tektek kontrol etmemızı ıstıyor
Ilk sekılde yanı 5 tane ozellıgı tek tek kontrol edeceksın ,scalar multıplıcatıon
da ıse 5 olan sm ozellıklerını tektek kullanarak bunun lınear space olup olmadıgını
kontrol edeceksın
3.soru
Burada bızden normal olan toplama ıslemını u+v yerıne uv seklınde tanımlayarak
Toplama ıslemının 5 ozellıgını saglayıp saglamadıgını kontrol etmemızı sonra 5 tane ca
Maya gore kontrol etmemızı ıstıyo sonra uc yide scalar multıplıcatıona gore kontrol
Etmemız ıstenıyor
4.soru
Bizim rkare deki bu sekılın ıcındekı 0 1 arasındakı x ve 0 bır arasındakı y nın altuzay olup olmadıgını
Kontrol etmemıızı ıstıyor
5.soru
Verılen polınomların subspace ( alt uzay ) olup olmadıgını tek tek gostermemizi istiyor bizden.
Kötü bir tercüme yapmışsınız. Birkaç çözüm yazabilirim.
Q4 için:
u,v∈W olacak şekilde u=(1/2,1/2) ve v=(1,1) seçelim. Allt uzay tanımı gereği toplamaya göre kapalı olmalıdır. u+v=(3/2,3/2)∉W olduğundan W, R2'nin alt vektör uzayı değildir.
Q3 için:
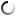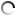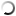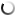
Q4 için:
u,v∈W olacak şekilde u=(1/2,1/2) ve v=(1,1) seçelim. Allt uzay tanımı gereği toplamaya göre kapalı olmalıdır. u+v=(3/2,3/2)∉W olduğundan W, R2'nin alt vektör uzayı değildir.
Q3 için:

Hocam ellerinize sağlık. Bunlarıda anlayıp çözebildiniz ya süpersiniz.
Hocam 1 ve 2 'yi kendim yaptım , 3 ve 4'ü sizin yaptığınızdan faydanalarak o mantığa göre tamamen kendim yaptım ve bitek 5.Sorum kalmıştı , onuda a ve c şıklarını bi arkadaştan aldım , b şıkkını yapmadan gönderdim.Tüm yardımlarınız için teşekkür ederim sayenizde 
Hocam ellerinize sağlık. Bunlarıda anlayıp çözebildiniz ya süpersiniz.
5. souda da iki polinom alıp toplamına ve çarpımına bakcaksınız verilen şartlar çerçevesinde zaten.
